- {x}
- Longest Common Subpath
- Erect the Fence II
- Count Square Sum Triples
- Nearest Exit from Entrance in Maze
- Sum Game
- Minimum Cost to Reach Destination in Time
- Concatenation of Array
- Unique Length-3 Palindromic Subsequences
- Painting a Grid With Three Different Colors
- Merge BSTs to Create Single BST
- Check if String Is Decomposable Into Value-Equal Substrings
- Confirmation Rate
- Maximum Number of Words You Can Type
- Add Minimum Number of Rungs
- Maximum Number of Points with Cost
- Maximum Genetic Difference Query
- Users That Actively Request Confirmation Messages
- Read More...

Painting a Grid With Three Different Colors
You are given two integers m and n. Consider an m x n grid where each cell is initially white. You can paint each cell red, green, or blue. All cells must be painted.
Return the number of ways to color the grid with no two adjacent cells having the same color. Since the answer can be very large, return it modulo 109 + 7.
Example 1:

Input: m = 1, n = 1 Output: 3 Explanation: The three possible colorings are shown in the image above.
Example 2:
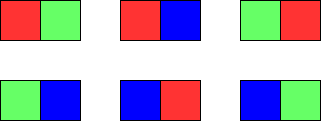
Input: m = 1, n = 2 Output: 6 Explanation: The six possible colorings are shown in the image above.
Example 3:
Input: m = 5, n = 5 Output: 580986
Constraints:
1 <= m <= 51 <= n <= 1000
Solution Explanation: Painting a Grid With Three Different Colors
This problem asks to find the number of ways to color an m x n grid with three colors (red, green, blue) such that no two adjacent cells have the same color. Because the answer can be very large, we need to use modulo arithmetic. The constraint 1 <= m <= 5 and 1 <= n <= 1000 suggests a dynamic programming approach with state compression.
Approach:
The core idea is to represent the coloring of each column as a state. Since there are m rows and 3 color choices per row, there are 3^m possible states for a single column. We can represent each state as an integer, where each digit in base 3 corresponds to the color of a row.
-
State Representation: A number
staterepresents a column's coloring. Each digit in the base 3 representation ofstatecorresponds to a row: 0, 1, and 2 represent red, green, and blue, respectively. For example, ifm = 3andstate = 5, the base 3 representation is120(because 5 = 13^2 + 23^1 + 0*3^0), representing the column with colors green, blue, and red in rows 1, 2, and 3, respectively. -
Validity Check: We need a function
f1(state)to check if astateis valid. Astateis invalid if any adjacent rows have the same color. This is easily checked by examining consecutive digits in the base 3 representation. -
Transition Function: We define
f2(prev_state, curr_state)to check if the transition fromprev_statetocurr_stateis valid. This means no two adjacent rows should have the same color across columns. This is also checked by comparing consecutive rows in base 3. -
Dynamic Programming: We use a DP array
dp[i][state]to store the number of ways to color the firsticolumns such that the last column is instate. The base case isdp[1][state] = 1ifstateis valid, otherwise 0. The recurrence relation is:dp[i][curr_state] = (sum of dp[i-1][prev_state] for all valid prev_state such that f2(prev_state, curr_state)) % mod -
Optimization: Since
dp[i]only depends ondp[i-1], we can use a rolling array of size 2 to save space. -
Final Answer: The final answer is the sum of all
dp[n][state]values for all valid statesstate.
Time and Space Complexity:
-
Time Complexity: O((m+n) * 3^(2m)). The outer loop iterates
ntimes. The inner loop iterates through all possible pairs of valid states, which is approximately3^m * 3^m. The validity checksf1andf2take O(m) time. -
Space Complexity: O(3^m). We use a DP array with a size proportional to the number of possible states for a column (3^m). The rolling array optimization reduces the space to O(3^m).
Code Explanation (Python):
The Python code implements the above algorithm efficiently using the described state representation, validity checks, and dynamic programming. The defaultdict efficiently handles the adjacency mapping between states. The % mod operation ensures we stay within the required modulo range.
The other languages (Java, C++, Go, TypeScript) follow the same logic with appropriate adaptations to their syntax and data structures. For example, Java uses HashSet and HashMap, while C++ employs unordered_set and unordered_map. Go utilizes built-in maps and slices. TypeScript employs Set and Map. The core algorithm remains consistent across all implementations.