You are given n BST (binary search tree) root nodes for n separate BSTs stored in an array trees (0-indexed). Each BST in trees has at most 3 nodes, and no two roots have the same value. In one operation, you can:

• Select two distinct indices i and j such that the value stored at one of the leaves of trees[i] is equal to the root value of trees[j].
• Replace the leaf node in trees[i] with trees[j].
• Remove trees[j] from trees.

Return the root of the resulting BST if it is possible to form a valid BST after performing n - 1 operations, or null if it is impossible to create a valid BST.

A BST (binary search tree) is a binary tree where each node satisfies the following property:

• Every node in the node's left subtree has a value strictly less than the node's value.
• Every node in the node's right subtree has a value strictly greater than the node's value.

A leaf is a node that has no children.

 

Example 1:

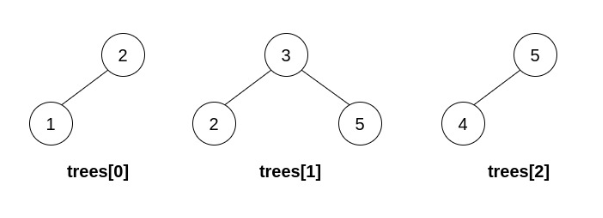
Input: trees = [[2,1],[3,2,5],[5,4]]
Output: [3,2,5,1,null,4]
Explanation:
In the first operation, pick i=1 and j=0, and merge trees[0] into trees[1].
Delete trees[0], so trees = [[3,2,5,1],[5,4]].

In the second operation, pick i=0 and j=1, and merge trees[1] into trees[0].
Delete trees[1], so trees = [[3,2,5,1,null,4]].

The resulting tree, shown above, is a valid BST, so return its root.

Example 2:

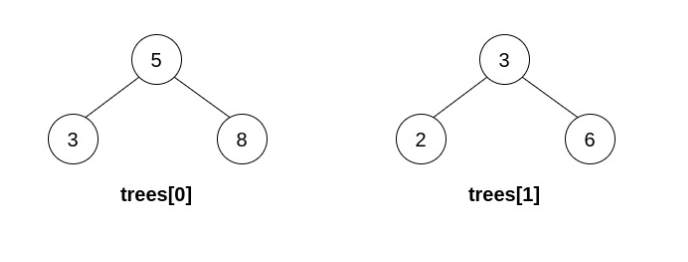
Input: trees = [[5,3,8],[3,2,6]]
Output: []
Explanation:
Pick i=0 and j=1 and merge trees[1] into trees[0].
Delete trees[1], so trees = [[5,3,8,2,6]].

The resulting tree is shown above. This is the only valid operation that can be performed, but the resulting tree is not a valid BST, so return null.

Example 3:

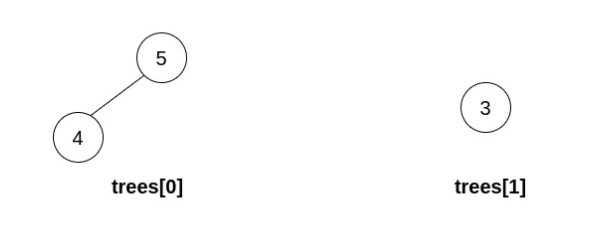
Input: trees = [[5,4],[3]]
Output: []
Explanation: It is impossible to perform any operations.

 

Constraints:

• n == trees.length
• 1 <= n <= 5 * 104
• The number of nodes in each tree is in the range [1, 3].
• Each node in the input may have children but no grandchildren.
• No two roots of trees have the same value.
• All the trees in the input are valid BSTs.
• 1 <= TreeNode.val <= 5 * 104.

class TreeNode:
    def __init__(self, val=0, left=None, right=None):
        self.val = val
        self.left = left
        self.right = right
 
def is_valid_bst(root):
    def helper(node, min_val, max_val):
        if not node:
            return True
        if not (min_val < node.val < max_val):
            return False
        return helper(node.left, min_val, node.val) and helper(node.right, node.val, max_val)
    return helper(root, float('-inf'), float('inf'))
 
def merge_bsts(trees):
    def solve(trees):
        if len(trees) == 1:
            return trees[0]
        for i in range(len(trees)):
            for j in range(len(trees)):
                if i == j:
                    continue
                
                tree1 = trees[i]
                tree2 = trees[j]
 
                #Attempt merges
                if merge_possible(tree1,tree2):
                   new_tree = merge_trees(tree1,tree2)
                   new_trees = [t for k,t in enumerate(trees) if k !=j]
                   new_trees[i] = new_tree
                   result = solve(new_trees)
                   if result:
                       return result
        return None
 
 
    def merge_possible(tree1, tree2):
      #Check leaves of tree1 against root of tree2
      q = [tree1]
      while q:
          curr = q.pop(0)
          if not curr.left and not curr.right and curr.val == tree2.val:
            return True
          if curr.left:
            q.append(curr.left)
          if curr.right:
            q.append(curr.right)
      return False
 
    def merge_trees(tree1,tree2):
      q = [tree1]
      while q:
          curr = q.pop(0)
          if not curr.left and not curr.right and curr.val == tree2.val:
              curr.left = tree2
              return tree1
          if curr.left:
            q.append(curr.left)
          if curr.right:
            q.append(curr.right)
      return None
 
 
    result = solve(trees)
    return result if is_valid_bst(result) else None