- {x}
- Prison Cells After N Days
- Check Completeness of a Binary Tree
- Regions Cut By Slashes
- Delete Columns to Make Sorted III
- N-Repeated Element in Size 2N Array
- Maximum Width Ramp
- Minimum Area Rectangle II
- Least Operators to Express Number
- Univalued Binary Tree
- Vowel Spellchecker
- Numbers With Same Consecutive Differences
- Binary Tree Cameras
- Pancake Sorting
- Powerful Integers
- Flip Binary Tree To Match Preorder Traversal
- Equal Rational Numbers
- K Closest Points to Origin
- Read More...
{x}

Univalued Binary Tree
A binary tree is uni-valued if every node in the tree has the same value.
Given the root of a binary tree, return true if the given tree is uni-valued, or false otherwise.
Example 1:
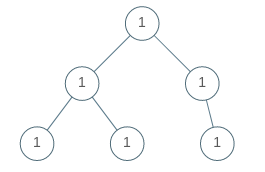
Input: root = [1,1,1,1,1,null,1] Output: true
Example 2:
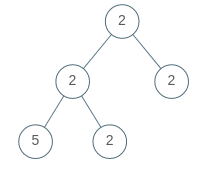
Input: root = [2,2,2,5,2] Output: false
Constraints:
- The number of nodes in the tree is in the range
[1, 100]. 0 <= Node.val < 100
965. Univalued Binary Tree
Problem Description
Determine if a given binary tree is univalued, meaning all nodes have the same value.
Solution Approach: Depth-First Search (DFS)
The most efficient approach is to use Depth-First Search (DFS). We recursively traverse the tree, checking if each node's value matches the root's value.
Algorithm:
- Base Case: If the current node is
null(empty), returntrue(empty subtrees are univalued). - Value Check: Check if the current node's value is equal to the root node's value (
x). If not, returnfalseimmediately. - Recursive Calls: Recursively call the DFS function on the left and right subtrees. The entire subtree is univalued only if both recursive calls return
true.
Time and Space Complexity:
- Time Complexity: O(N), where N is the number of nodes in the tree. We visit each node exactly once.
- Space Complexity: O(H), where H is the height of the tree. This is due to the recursive call stack. In the worst case (a skewed tree), H can be equal to N. In the best case (a balanced tree), H is log₂(N).
Code Implementation in Multiple Languages
Python
# Definition for a binary tree node.
# class TreeNode:
# def __init__(self, val=0, left=None, right=None):
# self.val = val
# self.left = left
# self.right = right
class Solution:
def isUnivalTree(self, root: Optional[TreeNode]) -> bool:
x = root.val # Store the root's value for comparison
def dfs(node):
if not node:
return True
if node.val != x:
return False
return dfs(node.left) and dfs(node.right)
return dfs(root)Java
/**
* Definition for a binary tree node.
* public class TreeNode {
* int val;
* TreeNode left;
* TreeNode right;
* TreeNode() {}
* TreeNode(int val) { this.val = val; }
* TreeNode(int val, TreeNode left, TreeNode right) {
* this.val = val;
* this.left = left;
* this.right = right;
* }
* }
*/
class Solution {
public boolean isUnivalTree(TreeNode root) {
int x = root.val;
return dfs(root, x);
}
private boolean dfs(TreeNode node, int x) {
if (node == null) return true;
if (node.val != x) return false;
return dfs(node.left, x) && dfs(node.right, x);
}
}C++
/**
* Definition for a binary tree node.
* struct TreeNode {
* int val;
* TreeNode *left;
* TreeNode *right;
* TreeNode() : val(0), left(nullptr), right(nullptr) {}
* TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
* TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
* };
*/
class Solution {
public:
bool isUnivalTree(TreeNode* root) {
int x = root->val;
return dfs(root, x);
}
bool dfs(TreeNode* node, int x) {
if (!node) return true;
if (node->val != x) return false;
return dfs(node->left, x) && dfs(node->right, x);
}
};Go
/**
* Definition for a binary tree node.
* type TreeNode struct {
* Val int
* Left *TreeNode
* Right *TreeNode
* }
*/
func isUnivalTree(root *TreeNode) bool {
x := root.Val
return dfs(root, x)
}
func dfs(node *TreeNode, x int) bool {
if node == nil {
return true
}
if node.Val != x {
return false
}
return dfs(node.Left, x) && dfs(node.Right, x)
}Javascript
/**
* Definition for a binary tree node.
* function TreeNode(val, left, right) {
* this.val = (val===undefined ? 0 : val)
* this.left = (left===undefined ? null : left)
* this.right = (right===undefined ? null : right)
* }
*/
/**
* @param {TreeNode} root
* @return {boolean}
*/
var isUnivalTree = function(root) {
const x = root.val;
function dfs(node) {
if (!node) return true;
if (node.val !== x) return false;
return dfs(node.left) && dfs(node.right);
}
return dfs(root);
};These code examples all implement the same DFS algorithm, demonstrating its concise and efficient nature for solving this problem. Remember to adapt the TreeNode definition according to your specific environment and language.
On This Page
Also Explore
DSA Questions
Prison Cells After N Days
DSA Questions
Check Completeness of a Binary Tree
DSA Questions
Regions Cut By Slashes
DSA Questions
Delete Columns to Make Sorted III
DSA Questions
N-Repeated Element in Size 2N Array
DSA Questions
Maximum Width Ramp
DSA Questions
Minimum Area Rectangle II
DSA Questions
Least Operators to Express Number
DSA Questions
Univalued Binary Tree
DSA Questions
Vowel Spellchecker
DSA Questions
Numbers With Same Consecutive Differences
DSA Questions
Binary Tree Cameras
DSA Questions
Pancake Sorting
DSA Questions
Powerful Integers
DSA Questions
Flip Binary Tree To Match Preorder Traversal
DSA Questions
Equal Rational Numbers
DSA Questions